Supercritical fluids, especially, water and carbon dioxide, have been studied for decades as the heat transfer media in power plant cycle, refrigeration, air-conditioning and heat pumps. Supercritical CO₂ is one of the working fluid considered for the next generation power plant, especially since CO₂ is non-flammable and non-toxic. Supercritical Brayton cycles were proposed because of its high potential for the development of high thermal efficiency power cycles. Brayton cycle using supercritical CO₂ as the working fluid operates above the critical pressure, enabling the cycle to utilize small back work for pumps due to the low specific volume near supercritical point with no phase change process. This reduces the cost of the power plant due to the elimination of the condenser and subsequent compactness in the power plant .

At pressures above critical pressure (7.38 MPa for CO₂), a transition from the liquid state to supercritical state occurs continuously as the temperature increases. The region above the critical pressure and temperature corresponds to supercritical region, where small fluid temperature variations near the critical point can lead to significant change in the thermophysical properties of the fluid. Within this region, the temperature at which the specific heat reaches its maximum represents the pseudocritical point for that operating pressure. Figure 1 shows examples of the thermal and transport properties of the carbon dioxide at different pressures above the critical pressure. The specific heat of the fluid in this region has a local peak at the pseudocritical temperature with the local peak value decreasing with increasing pressure (Fig. 1b). The other fluid properties (density, thermal conductivity and dynamic viscosity) also show significant variation within an extremely narrow temperature range near the pseudocritical region. A closely related, but not materialistic, property of fluids is their thermal convective property, which has a direct role on the design and performance of the thermal systems. The convective heat transfer coefficient of fluids at supercritical pressures has many outstanding features, and is remarkably different from that of subcritical pressure due to the rapid changes of thermophysical properties with temperature and pressure in this region.

Figure. 1 Variation of thermal and transport properties of CO₂ in the pseudocritical region at different pressures [NIST]



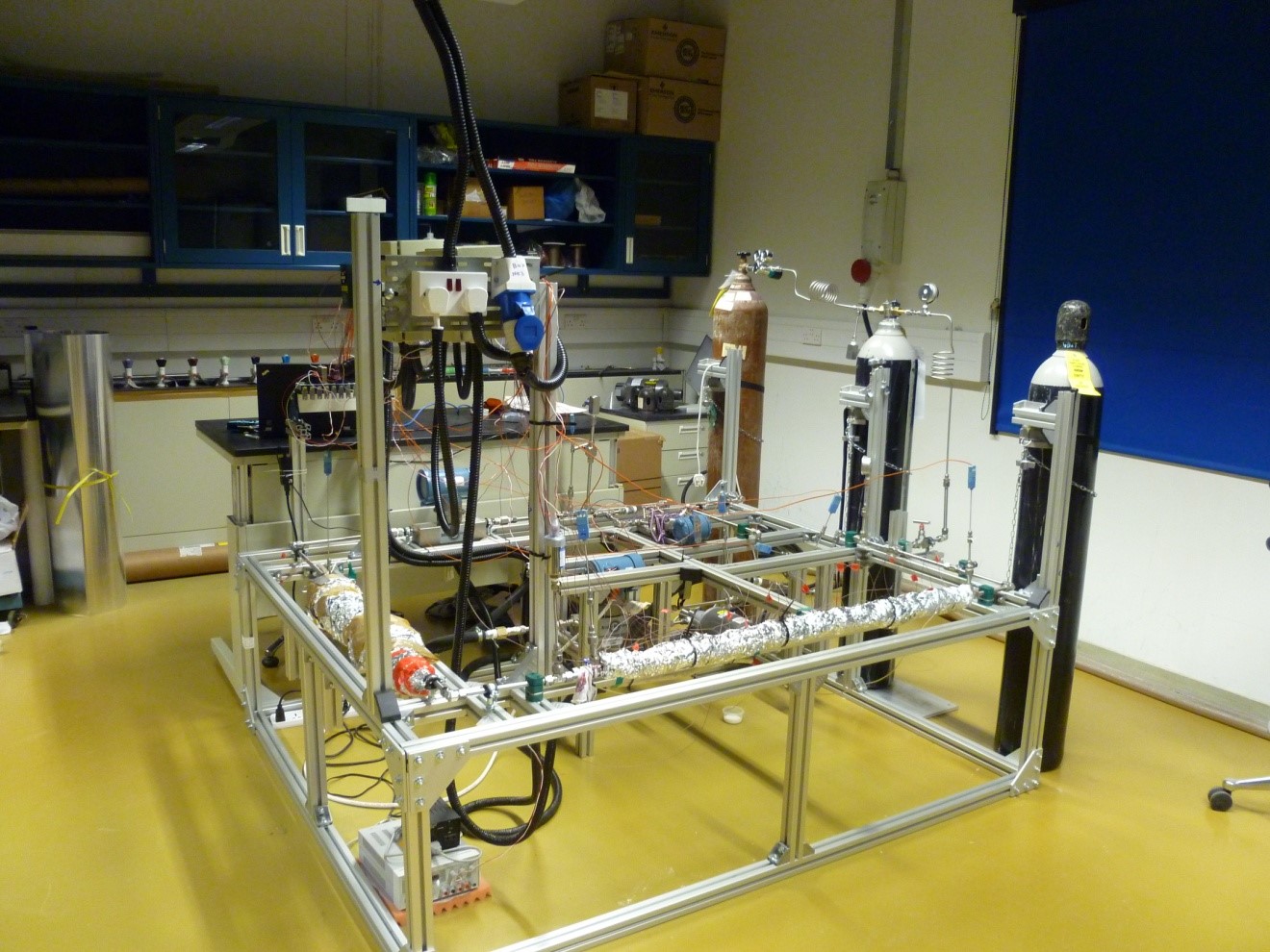
Figure. 2 Photograph of the Supercritical CO₂ facility in MSTF lab

Figure 2 shows schematics of this facility which consists of a high pressure CO₂ supply system, circular pump, cooler, flow meter, pre-heater, pressure transducers, thermocouples, tubes and valves. The system pressure, mass flow rate, inlet fluid temperature, and wall heat flux to the test section were measured and controlled as key experimental parameters using a NI-Labview DAQ system.  To achieve the experimental pressure, a CO₂ gas cylinder (purity 99.9 %) was pressurized by connecting it to a high pressure Helium gas cylinder, 15.0 MPa, through a high-pressure regulator. Experiments were carried out at different inlet temperatures and mass flow rates with constant system pressure and wall heat flux. Liquid CO₂ mass flow rate in the facility is maintained and precisely controlled using a magnetic driven gear pump (Micropump GLHH 25) and is measured using Coriolis mass flow meters (Micromotion F25 and Bronkhorst CORI-FLOW M54), and accuracy of 0.1 % (full scale). Fluid pressure at the inlet of the test section is measured using an absolute pressure transducer (Setra, Model 280E) with accuracy of 0.5 % (full scale). Fluid temperatures at the inlet and outlet of the test section are key experimental parameters because they are used for calculation of the fluid properties and bulk flow temperature throughout the test section. Inlet fluid temperature is monitored and adjusted to the desired value by a pre-heater which was designed and manufactured at the University of Wisconsin. The preheater consists of a simple double tube with a 5.5 kW heating element installed inside it. Several K-type thermocouple probes (Omega) were installed throughout the facility, before and after the test section, to monitor the temperatures of the working fluid in the facility. The bulk fluid temperature in the test section is measured at both the inlet and outlet using K-type thermocouple probes (Omega).

Figure. 3 Schematic of the facility and the cross-sectional view of the test-section

The test section is made of seamless stainless steel 316L tube with inner and outer diameters of 8.7 and 12.7 mm, respectively. The heated length of the test section is 1.14 m starting at 25 mm downstream of the test section inlet. The test section is heated by adding a constant heat flux to the outer surface of the tube via a total of 30 electric band heaters (MBH- 1215200B/120, OMEGA; each 200 W of power). The heater’s inner diameter is 32 mm and cylindrical copper blocks are placed between the tube and the heaters to conduct the heat flux from the heaters to the steel pipe. The outer walls of the band heaters were covered with thermal insulating materials to minimize the heat loss. The band heaters connected in a series of two, and the 15 pairs are then connected in parallel with a maximum heating power of 6 kW. Fourteen K-type thermocouple probes (SCASS-040U-3, OMEGA) were installed in 72 mm intervals along the test section to measure local wall temperatures, with the first thermocouple installed 72 mm downstream from the inlet of the test section. CO₂ flows through a double tube heat exchanger, is chilled with a cold water supply flow on the outer tube (1/2 in. diameter, stainless steel), while CO₂ flows through the inner tube (1/4 in. diameter, stainless steel) to return to the liquid state after passing through the test section. An independent water chiller which has a cooling capacity of 11 kW supplies cold water into the heat exchanger. During the test, all parameters such as temperatures, mass flow rate, and heating power were monitored and controlled by NI-Labview DAQ system.

Figure. 4 Schematic of the thermocouple arrangement in test-section

Figure 5 shows the measured heat transfer coefficient as a function of the fluid temperature normalized by pseudocritical temperature for the mass flow rate of 0.011 kg/s. The vertical lines on the symbols represent the 95% confidence interval uncertainties in the measurements. Uncertainty lines were displayed on few selected points in order to avoid the congestion of figures.

Figure. 5 Heat Transfer coefficient as a function of fluid temperature for mass flow rate of 0.011 kg/s

 

Effect of Buoyancy:

It is known that under forced convection in the high Reynolds number flow (Re_b ≈ 10⁵) the buoyancy effect might not be neglected even in a horizontal test section under heating conditions. In these cases, both the primary flow caused by the forced motion of the fluid and secondary flow caused by the buoyancy force must be considered for the calculation of heat transfer. This phenomenon has been investigated in detail for both the vertical and horizontal flow cases in the recent past.  Figure 6 presents the magnitude of the buoyancy parameter, Bu_c, as a function of the normalized distance from the pipe inlet. This figure shows that the buoyancy parameter Bu_c in the test section is about 10 times higher than the suggested limiting value for all the cases studied here, indicating the buoyancy force cannot be ignored anywhere in the test section. The value of Bu_c monotonically decreases along the pipe for all cases. Interestingly, Fig. 6a shows that as the wall heat flux increases, Bu_c decreases which can be attributed to the increase of the Reynolds number in the pipe due to the dramatic decrease of viscosity. Figure 6b shows the effect of the inlet pressure where for x/L < 0.25, Bu_c is within the uncertainty of the data for all the tested pressures. However, the buoyancy parameter at 7.5 MPa shows lower values than other pressures. The bulk fluid Reynolds number experiences a larger increase when the temperature reaches the corresponding pseudocritical temperature and hence, the magnitude of Bu_c becomes smaller in this region of flow compared to other inlet pressures. As expected, Fig. 6c shows that the buoyancy parameter, Bu_c, is higher for lower mass flow rates as it is inversely proportional to the Reynolds number. Overall, the current results indicate that the buoyancy parameter Bu_c points to the presence of buoyancy effects all through the test section. This is in agreement with the results reported in literature indicating the existence of buoyancy force with similar trends for Bu_c in horizontal circular pipe under high Reynolds number flow, albeit for a smaller diameter pipe. However, this does not present insight into the flow condition along the pipe as seen by the values of the wall convection coefficient.

 Figure. 6 Effect of the heat flux, inlet pressure, and mass flow rate on conventional buoyancy parameter Bu_c. Note that the limiting suggested value for neglecting buoyancy of Bu_c < 10^-3.

These results show that the common heat transfer correlation for turbulent pipe flow over predicts the Nusselt number near the critical temperature for all the flow conditions tested here. This is attributed to the inability of the considered correlation to properly account for the property variation of the fluid near the critical region. The effects of the buoyancy and fluid acceleration in the test section were investigated by considering three different buoyancy parameters. All criteria correctly indicated buoyancy effect is important in the present experiments. However, the results indicated that the conventional buoyancy parameter was more appropriate in predicting the importance of buoyancy for the experimental set up used in this work.

Selected Publications:

• Tanimizu, K., and Sadr, R., 2016, "Experimental investigation of buoyancy effects on convection heat transfer of supercritical CO2 flow in a horizontal tube," Heat and Mass Transfer, 52(4), pp. 713-726.
• Katsuyoshi Tanimizu, Reza Sadr, Devesh Ranjan, 2014, "Thermal and Hydraulic Characteristcis of SCO2 in a Horizontal tube at high Reynolds number," Proc. of the15th International Heat Transfer Conference, IHTC-15, IHTC, Kyoto, Japan, pp. IHTC15-8747.
• Katsuyoshi Tanimizu, and Reza Sadr, 2012, "Experimental Investigation of Heat Transfer Characteristics of SCO2 in a Circular Horizontal tube," ASME 2012 Summer Heat Transfer Conference, HT2012-58331, Puerto Rico, USA.

 

Supercritical Carbon dioxide

Upcoming project - CO2-based Alternative refrigerants

- Upcoming project (funded by Shell-Qatar): Performance investigation of alternative refrigerants (carbon dioxide-based blends) at fundamental (heat transfer characteristics) level and at refrigeration cycle level (vapor compression).

- More details will be updated soon.